How do bird nests stay together?

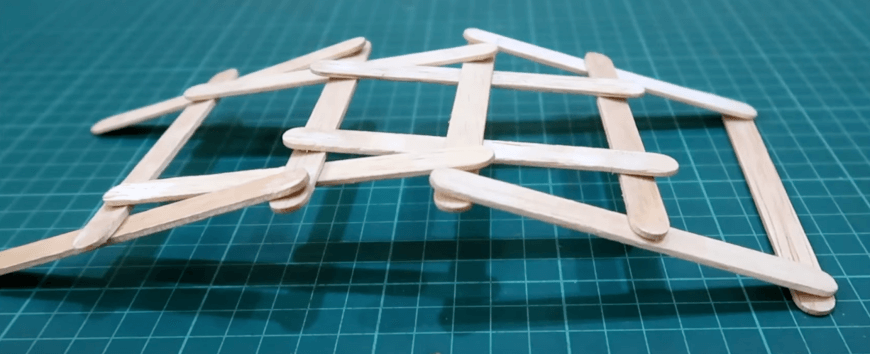
The concept of constructing a self-supporting structure made of rods — without the use of nails, ropes, or glue — dates back to Leonardo da Vinci. In the Codex Atlanticus, da Vinci illustrated a design for a self-supporting bridge across a river, which can be easily demonstrated using toothpicks, matches, or chopsticks. However, this design is fragile — pulling one of the rods or pushing the bridge from below can cause it to collapse.

In contrast, bird nests — which are also self-supporting structures consisting of rigid sticks and twigs — are remarkably stable despite continuous disturbances such as wind, ground vibrations, and the landing or takeoff of birds. What makes bird nests so sturdy?

This was the question at the center of a recent paper from L. Mahadevan and his team at the Harvard John A. Paulson School of Engineering and Applied Sciences (SEAS). Mahadevan is the Lola England de Valpine Professor of Applied Mathematics, of Organismic and Evolutionary Biology, and of Physics at SEAS and the Faculty of Arts and Sciences at Harvard. 

The research was supported in part by the National Science Foundation through the Harvard NSF MRSEC award.

While entanglement in small, flexible systems, such as polymers, is well understood, less is known about how stiff, macroscale components entangle, especially when they are densely packed.

“When we think about entanglement, we typically think about flexible, individual constituents wrapping around each other, as exemplified in tangled headphone cords or entangling vines,” said Mahadevan. “Contrary to this common intuition, stiff and straight rods can also entangle themselves – if they are long or thin enough.”

To understand how, the researchers used X-ray tomography — a technique that creates a detailed cross-section of an object — as well as computer simulation and experimentation to peer inside and reconstruct the complex structure of bird nests.

The team collaborated with the Harvard Museum of Comparative Zoology, which provided a real bird’s nest made from steel wires.

“Pigeons have been known to nest near construction sites and use scrap metal to make their nests, which worked out for us because X-ray scanning on metals provides a clear image to work with,” said Yeonsu Jung, a postdoctoral fellow in applied mathematics at SEAS and first author of the paper.

After imaging and mapping the real birds’ nests, the researchers created their own, using steel rods with varying length-to-diameter ratios, or aspect ratios. The research team found that the degree of entanglement within a pile of rods depended on this ratio. If the rods had a low aspect ratio — were too short and too wide — the entanglement would be weak and localized at separate spots. But rods with a high aspect ratio — were longer and thinner — had stronger entanglement throughout the entire structure.

“By looking inside these structures, we could see the percolations of entanglement,” said Jung. “For rods with a low aspect ratio, there could be pockets of entanglement, but those would still fall apart and stay unconnected. But for high aspect ratio rods, things are really connected inside and the nest would stay together.”

Unlike polymers and other microscopic filaments, the team also found that friction and gravity play a role in keeping these systems entangled as well. The team found that nests built with lower aspect ratio packing could become more entangled when exposed to force – in this case, being bounced up and down. 

The research sheds light not only on how bird nests stay together but also how other wonders of animal architecture function. It could also be used in the design and control of reconfigurable architectures or active fabrics.

“The role of local topological constraints, i.e. the inability of a filament to move through another filament in its vicinity, is what defines an ordered weave in a textile,” said Mahadevan.  “Our study of the random entanglement of stiff rods aimed to study how the same topological constraints operate in a system dominated by disorder. Simultaneously controlling both the disorder and topology to build functional nests efficiently is something that bird brains clearly find easy. But are we humans smart enough to decipher how they do so? Time will tell.”

This research was co-authored by Thomas Plumb-Reyes and Hao-Yu Greg Lin.